CBSE 10th Maths -  Triangles - Exercise 6.4

Solution:

Given, ΔABC ~ ΔDEF

Area of ΔABC = 64 $cm^2$

Area of ΔDEF = 121 $cm^2$

EF = 15.4 cm

$\dfrac{Area \: of \: angle \: \triangle ABC}{Area \: of \: angle \: \triangle DEF} = \dfrac{AB^2}{DE^2}$

As we know, if two triangles are similar, ratio of their areas are equal to the square of the ratio of their corresponding sides

⇒ $\dfrac{AC^2}{DF^2} = \dfrac{BC^2}{EF^2}$

$\dfrac{64}{121} = \dfrac{BC^2}{EF^2}$

$(\dfrac{8}{11})^2 = (\dfrac{BC}{15.4})^2$

$\dfrac{8}{11} = \dfrac{BC}{15.4}$

BC = 8×$\dfrac{15.4}{11}$

BC = 8 × 1.4

BC = 11.2 cm

Solution:

Given

ABCD is a trapezium with AB || DC. Diagonals AC and BD intersect each other at point O.

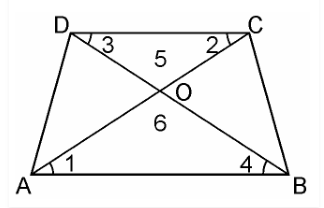
In ΔAOB and ΔCOD, we have

$\angle1 = \angle2$ (Alternate angles)

$\angle3 = \angle4$ (Alternate angles)

$\angle5 = \angle6$ (Vertically opposite angle)

∴ ΔAOB ~ ΔCOD [AAA similarity criterion]

As we know, If two triangles are similar then the ratio of their areas are equal to the square of the ratio of their corresponding sides.

Therefore,

$\dfrac{Area \: of \: (ΔAOB)}{Area \: of \: (ΔCOD} = \dfrac{AB^2}{CD^2}$

= $\dfrac{(2CD)^2}{CD^2}$ [∴ AB = 2CD]

∴ $\dfrac{Area \: of \: (ΔAOB)}{Area \: of \: (ΔCOD)}$

= $\dfrac{4CD^2}{CD^2} = \dfrac{4}{1}$

Hence, the required ratio of the area of ΔAOB and ΔCOD = 4:1

Solution:

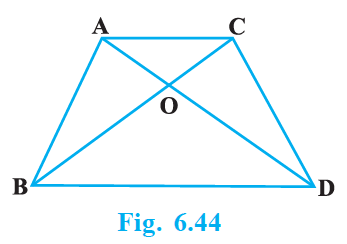
Given,

ABC and DBC are two triangles on the same base BC. AD intersects BC at O

We have to prove:

$\dfrac{Area (ΔABC)}{Area (ΔDBC)}$ = $\dfrac{AO}{DO}$

Let us draw two perpendiculars AP and DM on line BC

We know that area of a triangle = $\dfrac{1}{2}$ × Base × Height

$\dfrac{ar (\triangle ABC)}{ar(\triangle DEF)} = \dfrac{\frac{1}{2}\times BC \times AP}{\frac{1}{2}\times BC \times DM} = \dfrac{AP}{DM}$

In ΔAPO and ΔDMO

$\angle APO = \angle DMO$ (Each 90°)

$\angle AOP = \angle DOM$ (Vertically opposite angles)

∴ ΔAPO ~ ΔDMO (AA similarity criterion)

∴$\dfrac{AP}{DM} = \dfrac{AO}{DO}$

$\dfrac{Area (ΔABC)}{Area (ΔDBC)} = \dfrac{AO}{DO}$

Solution:

Say ΔABC and ΔPQR are two similar triangles and equal in area

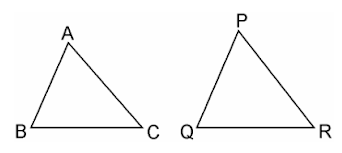
Now let us prove ΔABC ≅ ΔPQR.

Since, ΔABC ~ ΔPQR

$\dfrac{Area \: of \: (ΔABC)}{Area \: of \: (ΔPQR)} = \dfrac{BC^2}{QR^2}$

$\dfrac{BC^2}{QR^2} =1 [Since, Area(ΔABC) = (ΔPQR)$

$\dfrac{BC^2}{QR^2}$

BC = QR

Similarly, we can prove that

AB = PQ and AC = PR

Thus, ΔABC ≅ ΔPQR [SSS criterion of congruence]

Solution:

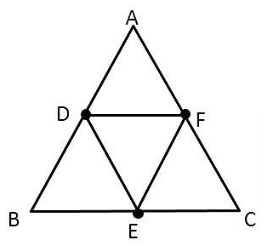
D, E, and F are the mid-points of ΔABC

DE || AC and

DE =$ \dfrac{1}{2}$ AC (Midpoint theorem) …. (1)

In ΔBED and ΔBCA

$\angle BED = \angle BCA$ (Corresponding angles)

$\angle BDE = \angle BAC$ (Corresponding angles)

$\angle EBD = \angle CBA$ (Common angles)

ΔBED∼ΔBCA (AAA similarity criterion)

$\dfrac{ar (ΔBED) }{ ar (ΔBCA)}=(\dfrac{DE}{AC})^2$

$\dfrac{ar (ΔBED) }{ ar (ΔBCA)} = \dfrac{1}{4}$ [From (1)]

ar (ΔBED) = $\dfrac{1}{4}$ ar (ΔBCA)

Similarly,

ar (ΔCFE) = $\dfrac{1}{4}$ ar (CBA) and ar (ΔADF) = $\dfrac{1}{4}$ ar (ΔADF) = $\dfrac{1}{4}$ ar (ΔABC)

Also,

ar (ΔDEF) = ar (ΔABC) − [ar (ΔBED) + ar (ΔCFE) + ar (ΔADF)]

ar (ΔDEF) = ar (ΔABC) − $\dfrac{3}{4}$ ar (ΔABC) = $\dfrac{1}{4}$ ar (ΔABC)

$\dfrac{ar (ΔDEF)}{ ar (ΔABC)} =\dfrac{1}{4}$

Solution:

Given:

AM and DN are the medians of triangles ABC and DEF respectively and ΔABC ~ ΔDEF

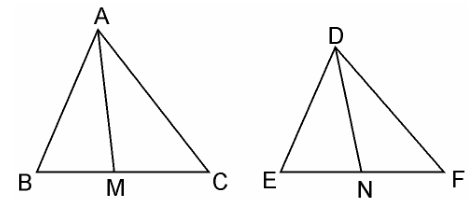
We have to prove: $\dfrac{Area(ΔABC)}{Area(ΔDEF)} = \dfrac{AM^2}{DN^2}$

Since, ΔABC ~ ΔDEF (Given)

$\dfrac{ Area(ΔABC)}{Area(ΔDEF)} = \dfrac{AB^2}{DE^2}$ ……………………………(i)

and,$\dfrac{AB}{DE} = \dfrac{BC}{EF} = \dfrac{CA}{FD} $………………………………………(ii)

$\dfrac{AB}{DE} = \dfrac{\frac{1}{2}\times BC}{\frac{1}{2}\times EF} = \dfrac{CD}{FD}$

In ΔABM and ΔDEN,

Since ΔABC ~ ΔDEF

$\angle B = \angle E$

$\dfrac{AB}{DE} = \dfrac{BM}{EN}$ [Already Proved in equation (i)]

ΔABC ~ ΔDEF [SAS similarity criterion]

$\dfrac{AB}{DE} = \dfrac{AM}{DN}$ …………………………………………………..(iii)

ΔABM ~ ΔDEN

As the areas of two similar triangles are proportional to the squares of the corresponding sides

$\dfrac{ area(ΔABC)}{area(ΔDEF)} = \dfrac{AB^2}{DE^2} = \dfrac{AM^2}{DN^2}$

Hence, proved

Solution:

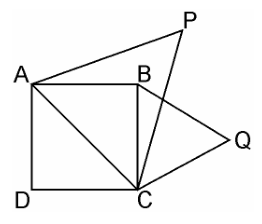
Given, ABCD is a square whose one diagonal is AC. ΔAPC and ΔBQC are two equilateral triangles described on the diagonals AC and side BC of the square ABCD.

Area(ΔBQC) = $\dfrac{1}{2}$ Area(ΔAPC)

Since, ΔAPC and ΔBQC are both equilateral triangles, as per given,

ΔAPC ~ ΔBQC [AAA similarity criterion]

$\dfrac{area(ΔAPC)}{area(ΔBQC)} = \dfrac{AC^2}{BC^2} = \dfrac{AC^2}{BC^2}$

Since, Diagonal = $\sqrt{2} side = \sqrt{2}$ BC = AC

area(ΔAPC) = 2 × area(ΔBQC)

area(ΔBQC) = $\dfrac{1}{2}$ area(ΔAPC)

Hence, proved