CBSE 10th Maths -  Triangles - Exercise 6.5

(i) 7 cm, 24 cm, 25 cm

Given, sides of the triangle are 7 cm, 24 cm, and 25 cm

Squaring the lengths of the sides of the, we will get 49, 576, and 625

49 + 576 = 625

$(7)^2 + (24)^2 = (25)^2$

Therefore, the above equation satisfies, Pythagoras theorem. Hence, it is right angled triangle

Length of Hypotenuse = 25 cm

(ii) 3 cm, 8 cm, 6 cm

Given, sides of the triangle are 3 cm, 8 cm, and 6 cm

Squaring the lengths of these sides, we will get 9, 64, and 36

Clearly, 9 + 36 ≠ 64

Or, $3^2 + 6^2 ≠ 8^2$

Therefore, the sum of the squares of the lengths of two sides is not equal to the square of the length of the hypotenuse

Hence, the given triangle does not satisfies Pythagoras theorem

(iii) 50 cm, 80 cm, 100 cm

Given, sides of triangle’s are 50 cm, 80 cm, and 100 cm

Squaring the lengths of these sides, we will get 2500, 6400, and 10000

However, 2500 + 6400 ≠ 10000

Or, $50^2 + 80^2 ≠ 100^2$

As you can see, the sum of the squares of the lengths of two sides is not equal to the square of the length of the third side

Therefore, the given triangle does not satisfies Pythagoras theorem

Hence, it is not a right triangle

(iv) 13 cm, 12 cm, 5 cm

Given, sides are 13 cm, 12 cm, and 5 cm

Squaring the lengths of these sides, we will get 169, 144, and 25

Thus, 144 +25 = 169

Or, $12^2 + 5^2 = 13^2$

The sides of the given triangle are satisfying Pythagoras theorem

Therefore, it is a right triangle

Hence, length of the hypotenuse of this triangle is 13 cm

Given, ΔPQR is right angled at P is a point on QR such that PM $\perp$ QR

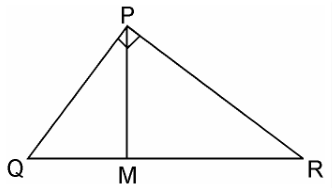
We have to prove, $PM^2$ = QM × MR

In ΔPQM, by Pythagoras theorem

$PQ^2 = PM^2 + QM^2$

Or, $PM^2 = PQ^2 – QM^2$ ……………………………..(i)

In ΔPMR, by Pythagoras theorem

$PR^2 = PM^2 + MR^2$

Or, $PM^2 = PR^2 – MR^2$ ………………………………………..(ii)

Adding equation, (i) and (ii), we get

$2PM^2 = (PQ^2 + PM^2) – (QM^2 + MR^2)$

= $QR^2 – QM^2 – MR^2 [∴ QR^2 = PQ^2 + PR^2]$

= $(QM + MR)^2 – QM^2 – MR^2$

= 2QM × MR

$PM^2$ = QM × MR

(i) $AB^2$ = BC . BD

In ΔADB and ΔCAB

$\angle DAB = \angle ACB $(Each 90°)

$\angle ABD = \angle CBA $(Common angles)

ΔADB ~ ΔCAB [AA similarity criterion]

$\dfrac{AB}{CB} = \dfrac{BD}{AB}$

$AB^2$ = CB × BD

(ii) $AC^2$ = BC . DC

Let $\angle CAB$ = x

In ΔCBA

$\angle CBA$ = 180° – 90° – x

$\angle CBA$ = 90° – x

Similarly, in ΔCAD

$\angle CAD = 90° – \angle CBA$

= 90° – x

$\angle CDA$ = 180° – 90° – (90° – x)

$\angle CDA$ = x

In ΔCBA and ΔCAD, we have

$\angle CBA = \angle CAD$

$\angle CAB = \angle CDA$

$\angle ACB = \angle DCA$ (Each 90°)

ΔCBA ~ ΔCAD [AAA similarity criterion]

$\dfrac{AC}{DC} = \dfrac{BC}{AC}$

$AC^2$ = DC × BC

(iii) $AD^2$ = BD . CD

In ΔDCA and ΔDAB

$\angle DCA = \angle DAB$ (Each 90°)

$\angle CDA = \angle ADB$ (common angles)

ΔDCA ~ ΔDAB [AA similarity criterion]

$\dfrac{DC}{DA} = \dfrac{DA}{DA}$

$AD^2$ = BD × CD

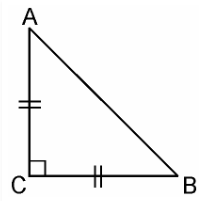
Given, ΔABC is an isosceles triangle right angled at C

In ΔACB, $\angle C$ = 90°

AC = BC (By isosceles triangle property)

$AB^2 = AC^2 + BC^2$ [By Pythagoras theorem]

= $AC^2 + AC^2$ [Since, AC = BC]

$AB^2 = 2AC^2$

Given, ΔABC is an isosceles triangle having AC = BC and $AB^2 = 2AC^2$

In ΔACB

AC = BC

$AB^2 = 2AC^2$

$AB^2 = AC^2 + AC^2$

= $AC^2 + BC^2$ [Since, AC = BC]

Hence, by Pythagoras theorem ΔABC is right angle triangle

Given, ABC is an equilateral triangle of side 2a

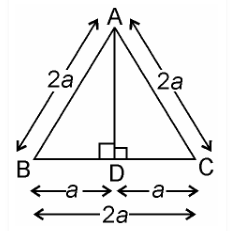
Draw, AD $\perp$ BC

In ΔADB and ΔADC

AB = AC

AD = AD

$\angle ADB = \angle ADC$ [Both are 90°]

Therefore, ΔADB ≅ ΔADC by RHS congruence

Hence, BD = DC [by CPCT]

In right angled ΔADB

$AB^2 = AD^2 + BD^2$

$(2a)^2 = AD^2 + a^2 $

$AD^2 = 4a^2 – a^2$

$AD^2 = 3a^2$

AD = $\sqrt{3a}$

Given, ABCD is a rhombus whose diagonals AC and BD intersect at O.

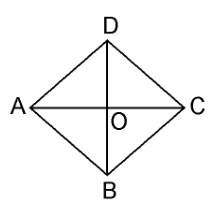
We have to prove, as per the question,

$AB^2 + BC^2 + CD^2 + AD^2 = AC^2 + BD^2$

Since, the diagonals of a rhombus bisect each other at right angles.

Therefore, AO = CO and BO = DO

In ΔAOB,

$\angle AOB$ = 90°

$AB^2 = AO^2 + BO^2$ …………………….. (i) [By Pythagoras theorem]

Similarly,

$AD^2 = AO^2 + DO^2$ …………………….. (ii)

$DC^2 = DO^2 + CO^2$ …………………….. (iii)

$BC^2 = CO^2 + BO2$ …………………….. (iv)

Adding equations (i) + (ii) + (iii) + (iv), we get,

$AB^2 + AD^2 + DC^2 + BC^2 = 2(AO^2 + BO^2 + DO^2 + CO^2)$

= $4AO^2 + 4BO^2$ [Since, AO = CO and BO =DO]

= $(2AO)^2 + (2BO)^2 = AC^2 + BD^2$

$AB^2 + AD^2 + DC^2 + BC^2 = AC^2 + BD^2$

Hence, proved.

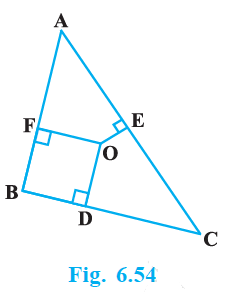
Given, in ΔABC, O is a point in the interior of a triangle

And OD $\perp$ BC, OE $\perp$ AC and OF $\perp$ AB

Join OA, OB and OC

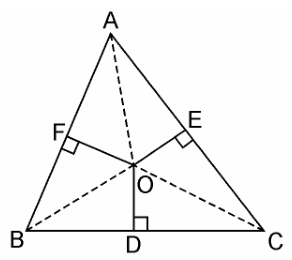
(i) $OA^2 + OB^2 + OC^2 – OD^2 – OE^2 – OF^2 = AF^2 + BD^2 + CE^2$

By Pythagoras theorem in ΔAOF, we have

$OA^2 = OF^2 + AF^2$

Similarly, in ΔBOD

$OB^2 = OD^2 + BD^2$

Similarly, in ΔCOE

$OC^2 = OE^2 + EC^2$

Adding these equations,

$OA^2 + OB^2 + OC^2 = OF^2 + AF^2 + OD^2 + BD^2 + OE^2 + EC^2$

$OA^2 + OB^2 + OC^2 – OD^2 – OE^2 – OF^2 = AF^2 + BD^2 + CE^2$

(ii) $AF^2 + BD^2 + EC^2 = (OA^2 – OE^2) + (OC^2 – OD^2) + (OB^2 – OF^2)$

$ AF^2 + BD^2 + CE^2 = AE^2 + CD^2 + BF^2$

Given, a ladder 10 m long reaches a window 8 m above the ground.

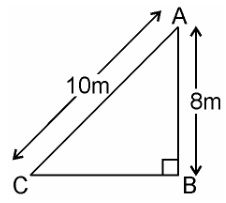
Let BA be the wall and AC be the ladder,

Therefore, by Pythagoras theorem,

$AC^2 = AB^2 + BC^2$

$10^2 = 8^2 + BC^2$

$BC^2$ = 100 – 64

$BC^2$ = 36

BC = 6m

Therefore, the distance of the foot of the ladder from the base of the wall is 6 m.

Given, a guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end

Let AB be the pole and AC be the wire

By Pythagoras theorem,

$AC^2 = AB^2 + BC^2$

$24^2 = 18^2 + BC^2$

$BC^2$ = 576 – 324

$BC^2$ = 252

BC = $6\sqrt{7}m$

Therefore, the distance from the base is $6\sqrt{7}$m