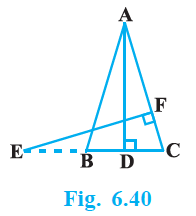
Given, ABC is an isosceles triangle.

AB = AC

$ \angle ABD = \angle ECF$

In ΔABD and ΔECF

$ \angle ADB = \angle EFC $(Each 90°)

$ \angle BAD = \angle CEF$ (Already proved)

ΔABD ~ ΔECF (using AA similarity criterion)

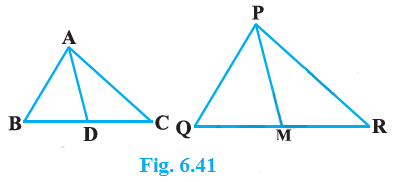
Given

ΔABC and ΔPQR, AB, BC and median AD of ΔABC are proportional to sides PQ, QR and median PM of ΔPQR

$\dfrac{AB}{PQ} = \dfrac{BC}{QR} = \dfrac{AD}{PM}$

We have to prove: ΔABC ~ ΔPQR

As we know here

$\dfrac{AB}{PQ} = \dfrac{BC}{QR} = \dfrac{AD}{PM}$

or $\dfrac{AB}{PQ} = \dfrac{(\dfrac{1}{2})BC}{(\dfrac{1}{2})QR} = \dfrac{AD}{PM}$

$\dfrac{AB}{PQ} = \dfrac{BC}{QR} = \dfrac{AD}{PM}$ (D is the midpoint of BC. M is the midpoint of QR)

ΔABD ~ ΔPQM [SSS similarity criterion]

$\angle ABD = \angle $PQM [Corresponding angles of two similar triangles are equal]

$\angle ABC = \angle PQR$

In ΔABC and ΔPQR

$\dfrac{AB}{PQ} = \dfrac{BC}{QR}$ ………………………….(i)

$\angle ABC = \angle PQR$ ……………………………(ii)

From equation (i) and (ii), we get

ΔABC ~ ΔPQR [SAS similarity criterion]

Given,

D is a point on the side BC of a triangle ABC such that $\angle ADC = \angle BAC$

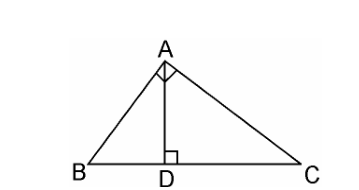
In ΔADC and ΔBAC

$\angle ADC = \angle BAC$ (Already given)

$\angle ACD = \angle BCA$ (Common angles)

ΔADC ~ ΔBAC (AA similarity criterion)

We know that corresponding sides of similar triangles are in proportion

$\dfrac{CA}{CB} = \dfrac{CD}{CA}$

$CA^2$ = CB.CD

Hence proved

Given,

Two triangles ΔABC and ΔPQR in which AD and PM are medians such that

$\dfrac{AB}{PQ} = \dfrac{AC}{PR} = \dfrac{AD}{PM}$

We have to prove, ΔABC ~ ΔPQR

Let us construct first

Produce AD to E so that AD = DE. Join CE, Similarly produce PM to N such that PM = MN, also Join RN.

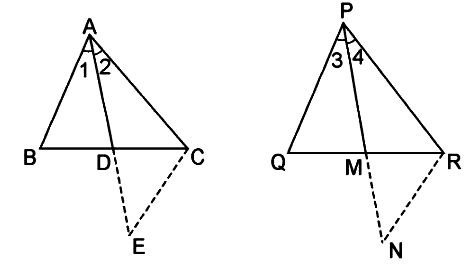
In ΔABD and ΔCDE, we have

AD = DE [By Construction.]

BD = DC [Since, AP is the median]

and, $\angle ADB = \angle CDE$ [Vertically opposite angles]

ΔABD ≅ ΔCDE [SAS criterion of congruence]

AB = CE [By CPCT] …………………………..(i)

Also, in ΔPQM and ΔMNR

PM = MN [By Construction]

QM = MR [Since, PM is the median]

and, $\angle PMQ = \angle NMR $[Vertically opposite angles]

ΔPQM = ΔMNR [SAS criterion of congruence]

PQ = RN [CPCT] ………………………………(ii)

Now, $\dfrac{AB}{PQ} = \dfrac{AC}{PR} = \dfrac{AD}{PM}$

From equation (i) and (ii)

$\dfrac{CE}{RN} = \dfrac{AC}{PR} = \dfrac{AD}{PM}$

$\dfrac{CE}{RN} = \dfrac{AC}{PR} = \dfrac{2AD}{2PM}$

$\dfrac{CE}{RN} = \dfrac{AC}{PR} = \dfrac{AE}{PN}$ [Since 2AD = AE and 2PM = PN]

ΔACE ~ ΔPRN [SSS similarity criterion]

Therefore,$ \angle 2 = \angle 4$

Similarly,$ \angle 1 = \angle 3$

$\angle 1 + \angle 2 = \angle 3 + \angle 4$

$\angle A = \angle P$ …………………………………………….(iii)

Now, in ΔABC and ΔPQR, we have

$\dfrac{AB}{PQ} = \dfrac{AC}{PR}$ (Already given)

From equation (iii)

$\angle A = \angle P$

ΔABC ~ ΔPQR [ SAS similarity criterion]

Given,

Length of the vertical pole = 6m

Shadow of the pole = 4 m

Let Height of tower = h m

Length of shadow of the tower = 28 m

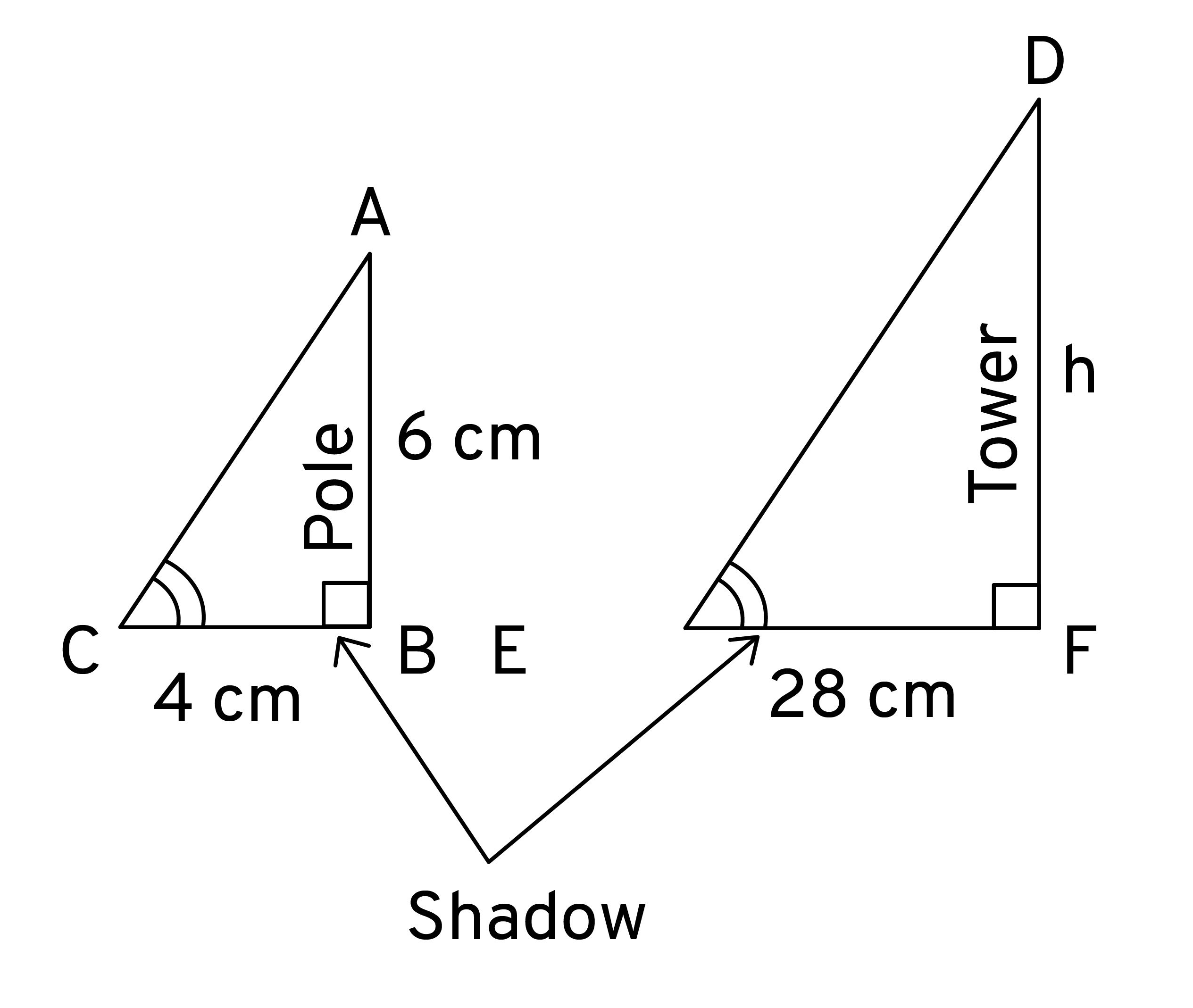
In ΔABC and ΔDEF

$\angle C = \angle E$ (angular elevation of sum)

$\angle B = \angle F = 90°$

ΔABC ~ ΔDEF (AA similarity criterion)

$\dfrac{AB}{DF} = \dfrac{BC}{EF}$ (If two triangles are similar corresponding sides are proportional)

$\dfrac{6}{h} = \dfrac{4}{28}$

$h = \dfrac{(6×28)}{4}$

h = 6 × 7

h = 42 m

Hence, the height of the tower is 42 m.

Given, ΔABC ~ ΔPQR

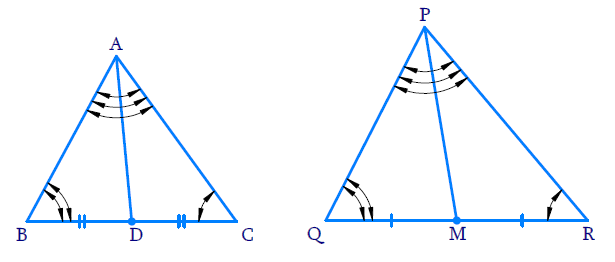
We know that the corresponding sides of similar triangles are in proportion.

$\dfrac{AB}{PQ} = \dfrac{AC}{PR} = \dfrac{BC}{QR} $……………………………(i)

Also, $\angle A = \angle P, \angle B = \angle Q, \angle C = \angle R $………….…..(ii)

Since AD and PM are medians, they will divide their opposite sides.

$BD = \dfrac{BC}{2}$ and $QM = \dfrac{QR}{2} $……………..………….(iii)

From equations (i) and (iii), we get

$\dfrac{AB}{PQ} = \dfrac{BD}{QM} $……………………….(iv)

In ΔABD and ΔPQM

From equation (ii), we have

$\angle B = \angle Q$

From equation (iv), we have

$\dfrac{AB}{PQ} = \dfrac{BD}{QM}$

ΔABD ~ ΔPQM (SAS similarity criterion)

$\dfrac{AB}{PQ} = \dfrac{BD}{QM} = \dfrac{AD}{PM}$